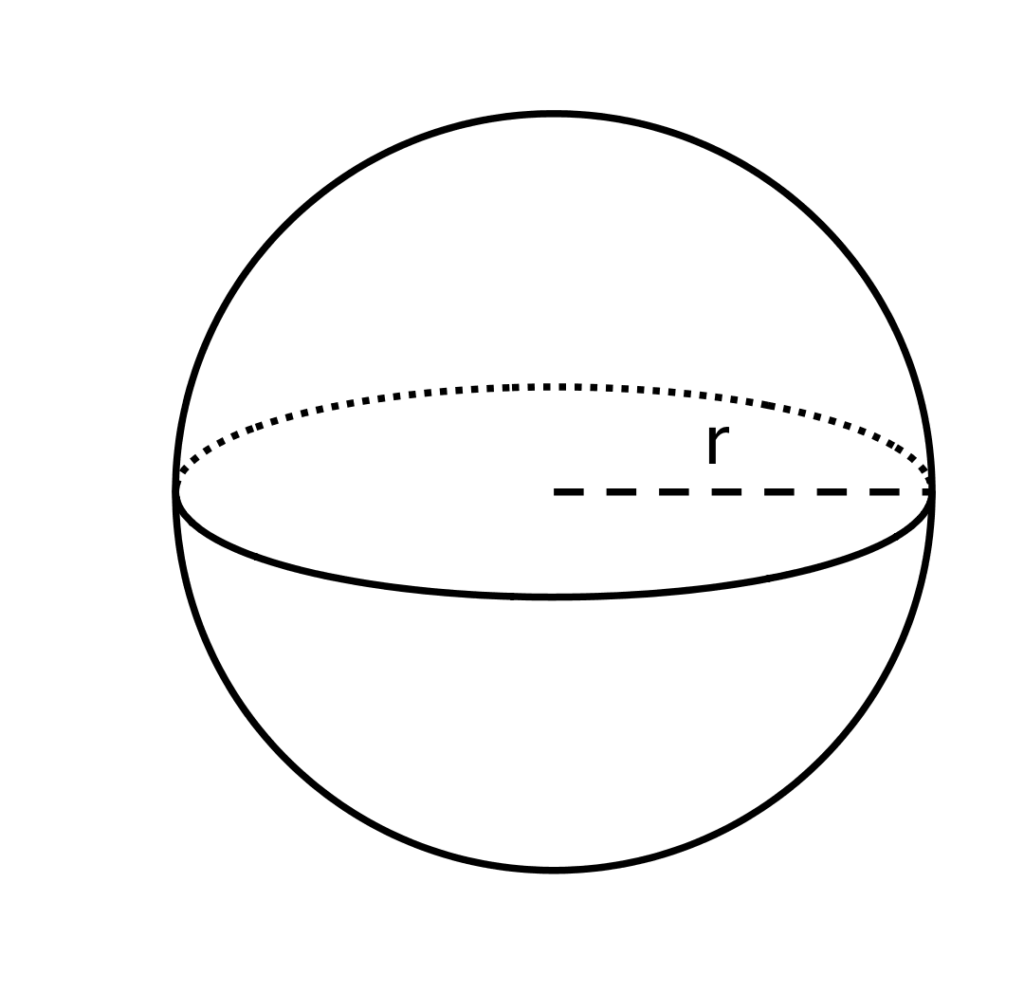
As with triangles and rectangles, we can attempt to derive formulas for the area and "perimeter" of a circle. Calculating the circumference of a circle is not as easy as calculating the perimeter of a rectangle or triangle, however. A circle is a geometric form of which every point on the outside of the circle is the same distance away from the center. The distance around the edge of the circle is called the circumference.
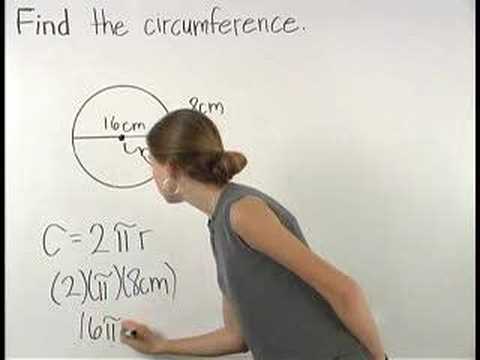
The distance from one side of the circle to the other, going through the center of the circle, is the diameter. The constant pi, designated by the Greek letter π, is the ratio of the circumference to the diameter of a circle. For any circle, if you divide the circumference by the diameter you get pi, an irregular number usually rounded to 3.14. The circumference of a circle is the measurement around a circle's edge. It can be compared to finding the perimeter of a shape . If you were to cut a circle and lay the outline flat, the length of the line it created would be its circumference.
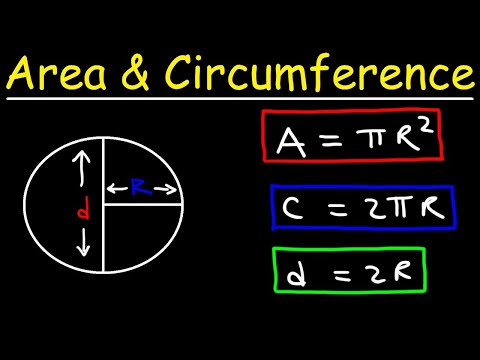
Whichever unit the radius is measured in will also be the unit the circumference is calculated in. The diameter is two times the radius, so the equation for the circumference of a circle using the radius is two times pi times the radius. If you are given the circumference but not the radius or the diameter, you can still solve for one or the other using these formulas. A circle is a two-dimensional shape made by drawing a curve that is the same distance all around from the center. The circumference of a circle of radius $r$ is $2\pi r$. This well known formula is taken up here from the point of view of similarity.
It is important to note in this task that the definition of $\pi$ already involves the circumference of a circle, a particular circle. In order to show that the ratio of circumference to diameter does not depend on the size of the circle, a similarity argument is required. Two different approaches are provided, one using the fact that all circles are similar and a second using similar triangles.
This former approach is simpler but the latter has the advantage of leading into an argument for calculating the area of a circle. To understand how to calculate circumference we must first begin with the definition of circumference. Circumference of a circle is linear distance around outer border of a circle. To find out the circumference, we need to know its diameter which is the length of its widest part. The diameter should be measured in feet for square footage calculations and if needed, converted to inches , yards , centimetres , millimetres and metres .
The circumference of a circle is its perimeter or distance around it. It is denoted by C in math formulas and has units of distance, such as millimeters, centimeters, meters, or inches. The circumference of a circle is the measured total length around a circle, which when measured in degrees is equal to 360°.
The "°" is the mathematical symbol for degrees. Two formulas are used to find circumference, C, depending on the given information. Both circumference formulas use the irrational number Pi, which is symbolized with the Greek letter, π. Pi is a mathematical constant and it is also the ratio of the circumference of a circle to the diameter. Use the circle circumference formula to calculate the circumference of the following circles. The diameter of a circle is twice to that of the radius.
If the diameter or radius of a circle is given, then we can easily find the circumference. We can also find the diameter and radius of a circle if the circumference is given. We round off to 3.14 in order to simplify our calculations. Circumference, diameter and radii are calculated in linear units, such as inches and centimeters. A circle has many different radii and many different diameters, and each one passes through the center.
Does calculating circumference have you running in circles? Our circumference calculator is an easy way for you to find the circumference of any circular object. The radius, the diameter, and the circumference are the three defining aspects of every circle. Given the radius or diameter and pi you can calculate the circumference.
The diameter is the distance from one side of the circle to the other at its widest points. The diameter will always pass through the center of the circle. You can also think of the radius as the distance between the center of the circle and its edge. In above program, we first take radius of circle as input from user and store it in variable radius.
Then we calculate the circumference of circle using above mentioned formulae and print it on screen using cout. The diameter of a circle, by contrast, is the longest distance from one edge of the circle to the opposite edge. The diameter is a special type of chord, a line that joins any two points of a circle.
The diameter is twice as long as the radius, so if the radius is 2 inches, for example, the diameter would be 4 inches. If the radius is 22.5 centimeters, the diameter would be 45 centimeters. Think of the diameter as if you are cutting a perfectly circular pie right down the center so that you have two equal pie halves. The line where you cut the pie in two would be the diameter.
Only a few of these measurements involve straight lines, so you need to know both the formulas and units of measurement required for each. Therefore, to calculate the circumference of a circle, we apply a formula that uses the radius or the diameter of the circle and the value of Pi (π). This means that the circumference is always about 3.14 (π) times the diameter . The formula below allows you to easily calculate the circumference of a circle when you know its diameter.
To find the circumference of a circle, multiply π times the diameter of the circle. Thus, a circle is simply the set of all points equidistant from a center point . The distance r from the center of the circle to the circle itself is called the radius; twice the radius is called the diameter. The radius and diameter are illustrated below.
Not just this but there are some significant distances on a circle that needs to be calculated before finding the circumference of the circle. Diameter is the distance from one side of the circle to the other, crossing through the center/ middle of the circle. As stated before, the perimeter or circumference of a circle is the distance around a circle or any circular shape. The circumference of a circle is the same as the length of a straight line folded or bent to make the circle. The circumference of a circle is measured in meters, kilometers, yards, inches, etc. The distance around a polygon, such as a square or a rectangle, is called the perimeter .
On the other hand, the distance around a circle is referred to as the circumference . Therefore, the circumference of a circle is the linear distance of an edge of the circle. Once again in this example, we're given the radius of the circle.
Although it's not a clean number like our previous example, but we can still simply plug the number directly into the formula like what we did above. Be aware of the units that this circle's radius is given in and remember to give your final answer in the same unit. In this question, we find that the circumference is equalled to 53.41m.
The distance around a rectangle or a square is as you might remember called the perimeter. The distance around a circle on the other hand is called the circumference . Lauren is planning her trip to London, and she wants to take a ride on the famous ferris wheel called the London Eye. While researching facts about the giant ferris wheel, she learns that the radius of the circle measures approximately 68 meters. What is the approximate circumference of the ferris wheel?
Pi is a constant value used for the measurement of the area and circumference of a circle or other circular figures. The symbol of pi is π and its numeric value is equal to 22/7 or 3.14. Further, these numeric values are used based on the context of the equation. The perimeter of a circle is the same as the circumference of a circle. It is the total length of the outer boundary of the circle. The perimeter or circumference of a circle is the product of the constant 'pi' and the diameter of the circle.
It is expressed in linear units like m, inch, cms, feet. You can think of it as the line that defines the shape. For shapes made of straight edges this line is called theperimeter but for circles this defining line is called the circumference. The distance from the centre to the outer line of the circle is called a radius.
It is the most important quantity of the circle based on which formulas for the area and circumference of the circle are derived. Twice the radius of a circle is called the diameter of the circle. The diameter cuts the circle into two equal parts, which is called a semi-circle. When we use the formula to calculate the circumference of the circle, then the radius of the circle is taken into account. Hence, we need to know the value of the radius or the diameter to evaluate the perimeter of the circle.
Circumference of the circle or perimeter of the circle is the measurement of the boundary of the circle. Whereas the area of circle defines the region occupied by it. If we open a circle and make a straight line out of it, then its length is the circumference. It is usually measured in units, such as cm or unit m. Diameter radius circumference The perimeter of a circle is called the circumference .
Its diameter is 57.28 feet the formula of circumference of circle is 2 pi r , from this calculate... Along the way, you also learned a little geography and history, which may also come in handy to you. This first argument is an example of MP7, Look For and Make Use of Structure.
The key to this argument is identifying that all circles are similar and then applying the meaning of similarity to the circumference. The second argument exemplifies MP8, Look For and Express Regularity in Repeated Reasoning. Here the key is to compare the circle to a more familiar shape, the triangle. The first solution requires a general understanding of similarity of shapes while the second requires knowledge of similarity specific to triangles. Worksheetto calculate circumference of circle when given diameter or radius.
The center of a circle is a point that's the same distance from any point on the circle itself. This distance is called the radius of the circle, or r for short. And any line segment from one point on the circle through the center to another point on the circle is called a diameter, or d for short. Thus, we can calculate the circumference of a circle if we know the circle's radius . For most calculations that require a decimal answer, estimating π as 3.14 is often sufficient.
For instance, if a circle has a radius of 3 meters, then its circumference C is the following. It looks like you calculated the area of a circle using a radius of 2; in this figure, the radius of each circle is 1. To find the area of the figure, imagine the two semi-circles are put together to create one circle. Then calculate the area of the circle and add it to the area of the square.
Now that you know how to calculate the circumference and area of a circle, you can use this knowledge to find the perimeter and area of composite figures. A circle represents a set of points, all of which are the same distance away from a fixed, middle point. The distance from the center of the circle to any point on the circle is called the radius. The Greek letter p (pronounced as "pie") is used to describe this number. It stands for the ratio between the circumference of any circle and its diameter, and it's true for all circles.
This means that any circle's circumference will be about 3.14 times the length of its diameter. Now to practice, try drawing a circle on a piece of paper, and measure your diameter with a ruler. The circumference of a circle is the perimeter of the circle. It is the total length of the boundary of the circle.
The circumference of a circle is the product of the constant π and the diameter of the circle. A person walking across a circular park, or a circular table to be bordered requires this metric of the circumference of a circle. The circumference is a linear value and its units are the same as the units of length. Pi (π) is a special mathematical constant; it is the ratio of circumference to diameter of any circle. The circumference is the distance around the circle. The diameter is a straight line that passes through the center of the circle.
It starts from a point on the circle, and ends at the center of the circle. Half of the diameter, or the distance from the midpoint to the circle border, is called the radius of the circle . Circumference is in essence the distance or length around a circle. _, correctly pronounced like pie, is an irrational number, which means that it cannot be written as a fraction. So to be sure, a good approximation of π is 3.14 when used in particular formulas. The length of wire needed for each paper clip is the total length of all the straight sections and the arc length of the three semi circles .